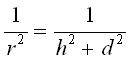Italian version
[Question]
Answer
Things are a little bit more complex with regards to the question.
It's possible to read an online summary of Haselhoff's claims here:
http://www.dcccs.org/bols.htm
It is possible to download Haselhoff's paper (which was published in a scientific peer-review journal "Physiolgia Plantarum") from here:
http://www.ecn.org/cunfi/Haselhoff.pdf
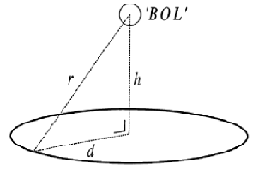
With reference to figure 1 shown in the paper, the equation written in the question is correct without any doubt.
The Pythagoras' Theorem claims that the sum of (areas of) the two small squares (h² + d²) equals the area of the larger one (r²), so:
(r²) = (h² + d²)
then, easily:
1/(r²) = 1/ (h² + d²)
which agrees with the formula written in the question.
Furthermore it's true that the intensity of an electromagnetic point source decays proportionally to 1/(r²).
According to Haselhoff, Balls Of Light (BOL) would be involved during the formation of a crop circle, irradiating the crop in the fields and producing a node stem lengthening proportional to 1/(r²), assuming the BOL being an electromagnetic point source.
Besides, Crop Circles Made by "Balls of Light" is the title of Haselhoff's web article, but this persuades any reader to believe that he demonstrated crop circles were made by BOLs, in other words that BOLs could flatten wheat or cereal stems to the ground. This is absolutely not demonstrated, nor it seems plausible.
Please consult FAQs on the CICAP web section:
http://www.cicap.org/crops/
In confirmation of this, Haselhoff claims on the web page conclusions that "My paper [...] nor does it explain how the crop is flattened". This directly contradicts the title of his paper which implicitly states that BOLs could flatten wheat or cereal stems to the ground.
So, we can better describe what Haselhoff claims as follows :
- in genuine crop circles node lengthening is proportional to 1/(r²), showing therefore a possible relation with the fact that during the crop circles formation an irradiating BOL could be present over the field;
- this statistical relationship is not evident in crop circles made by men with simple flattening tools (planks, ropes, stakes etc.).
Now the reader could be persuaded that he had found an absolute criterion in order to establish without any doubt if a crop circle has been made by men with simple flattening tools or not.
One more time, Haselhoff helps us claiming in the paper conclusion that: "By no means does the author pretend to present a 'lithmus test' for distinction between a genuine crop formation, whatever it may be, and a hand-flattened area of crop."
In other words, despite the strong statistical association presented by Haselhoff, by his own admission it is not absolutely able to determine if a crop circle has been made by men with simple flattening tools or not.
For this reason, it is possible to enter a man made formation, pick up the stems, measure the node length and find at the end the same statistical correlation presented by Haselhoff in his paper. If that happened, it would be consistent with Haselhoff's claim.
To sum up, the equation is right, but Haselhoff's claims don't prove anything at all, nor do they give a criterion to answer the crucial question:
- how can we objectively establish if a crop circle has been made by men with simple flattening tools or not?
This topic is widely discussed on the CICAP web section dedicated to crop circles
by Francesco Grassi
CICAP
Comitato Italiano per il Controllo delle Affermazioni sul Paranormale
[Question]
Could you explain if the following equation is right or not ? :
Eltjo Haselhoff PhD claims this equation is the explanation of the crop circle phenomenon. But I'm a little bit sceptical and I would like to understand more about this.
(Note: h is the height of a Ball Of Light from the ground; d is the reference distance in order to establish the irradiated energy intensity.
Question by: Cinzia Di Stasi
Received: 19 October 2003
Eltjo Haselhoff PhD claims this equation is the explanation of the crop circle phenomenon. But I'm a little bit sceptical and I would like to understand more about this.
(Note: h is the height of a Ball Of Light from the ground; d is the reference distance in order to establish the irradiated energy intensity.
Question by: Cinzia Di Stasi
Received: 19 October 2003
Answer
Things are a little bit more complex with regards to the question.
It's possible to read an online summary of Haselhoff's claims here:
http://www.dcccs.org/bols.htm
It is possible to download Haselhoff's paper (which was published in a scientific peer-review journal "Physiolgia Plantarum") from here:
http://www.ecn.org/cunfi/Haselhoff.pdf
With reference to figure 1 shown in the paper, the equation written in the question is correct without any doubt.
The Pythagoras' Theorem claims that the sum of (areas of) the two small squares (h² + d²) equals the area of the larger one (r²), so:
(r²) = (h² + d²)
then, easily:
1/(r²) = 1/ (h² + d²)
which agrees with the formula written in the question.
Furthermore it's true that the intensity of an electromagnetic point source decays proportionally to 1/(r²).
According to Haselhoff, Balls Of Light (BOL) would be involved during the formation of a crop circle, irradiating the crop in the fields and producing a node stem lengthening proportional to 1/(r²), assuming the BOL being an electromagnetic point source.
Besides, Crop Circles Made by "Balls of Light" is the title of Haselhoff's web article, but this persuades any reader to believe that he demonstrated crop circles were made by BOLs, in other words that BOLs could flatten wheat or cereal stems to the ground. This is absolutely not demonstrated, nor it seems plausible.
Please consult FAQs on the CICAP web section:
http://www.cicap.org/crops/
In confirmation of this, Haselhoff claims on the web page conclusions that "My paper [...] nor does it explain how the crop is flattened". This directly contradicts the title of his paper which implicitly states that BOLs could flatten wheat or cereal stems to the ground.
So, we can better describe what Haselhoff claims as follows :
- in genuine crop circles node lengthening is proportional to 1/(r²), showing therefore a possible relation with the fact that during the crop circles formation an irradiating BOL could be present over the field;
- this statistical relationship is not evident in crop circles made by men with simple flattening tools (planks, ropes, stakes etc.).
Now the reader could be persuaded that he had found an absolute criterion in order to establish without any doubt if a crop circle has been made by men with simple flattening tools or not.
One more time, Haselhoff helps us claiming in the paper conclusion that: "By no means does the author pretend to present a 'lithmus test' for distinction between a genuine crop formation, whatever it may be, and a hand-flattened area of crop."
In other words, despite the strong statistical association presented by Haselhoff, by his own admission it is not absolutely able to determine if a crop circle has been made by men with simple flattening tools or not.
For this reason, it is possible to enter a man made formation, pick up the stems, measure the node length and find at the end the same statistical correlation presented by Haselhoff in his paper. If that happened, it would be consistent with Haselhoff's claim.
To sum up, the equation is right, but Haselhoff's claims don't prove anything at all, nor do they give a criterion to answer the crucial question:
- how can we objectively establish if a crop circle has been made by men with simple flattening tools or not?
This topic is widely discussed on the CICAP web section dedicated to crop circles
by Francesco Grassi
CICAP
Comitato Italiano per il Controllo delle Affermazioni sul Paranormale